GLE Example: wulfnet.gle

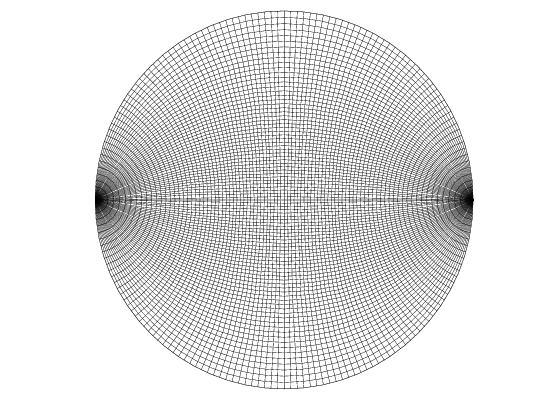
[PDF file]

 

---

! This example was provided by Ole Göbel

size 29 21

rp = 10                                            ! radius of primitive, only value that needs to be changed to blow up
f = pi/180                                         ! or shrink the entire net
thinl = 0.005                                      ! lwidth of thin lines 
thickl = 0.01                                      ! lwidth of thick lines

!drawing primitive and central cross
set lwidth .02
amove 15 10.5
circle rp
rmove 0 -rp
rline 0 2*rp
rmove -rp -rp
rline 2*rp 0
rmove -rp 0

!drawing small circles around east pole
for ang=2 to 88 step 2                             ! defining the grid of small circles
   set lwidth thinl                                ! ang must not become 0 nor 90!
   ang2=180-ang
   r1=rp*sin(ang*f)/(1+cos(ang*f))
   r2=rp*sin(ang2*f)/(1+cos(ang2*f))
   om=(r1+r2)/2
   r=r2-om
   rmove om 0
   if ang/10=int(ang/10) then
      set lwidth thickl                            ! drawing every small circle fatter with: angular
   end if                                          ! distance to east pole = n*10deg
   arc r 180-ang 180+ang
   rmove -om 0
next ang

!drawing small circles around west pole
for ang=2 to 88 step 2                             ! defining the grid of small circles
   set lwidth thinl                                ! ang must not become 0 nor 90!
   ang2=180-ang
   r1=rp*sin(ang*f)/(1+cos(ang*f))
   r2=rp*sin(ang2*f)/(1+cos(ang2*f))
   om=(r1+r2)/2
   r=r2-om
   rmove -om 0
   if ang/10=int(ang/10) then
      set lwidth thickl                            ! drawing every small circle fatter with: angular
   end if                                          ! distance to west pole = n*10deg
   arc r -ang ang
   rmove om 0
next ang

!drawing great circles in northern hemisphere
for ang=2 to 88 step 2                             ! defining the grid of great circles
   set lwidth thinl                                ! ang must not become 0 nor 90!
   ang2=180-ang
   r1=rp*sin(ang*f)/(1+cos(ang*f))
   r2=rp*sin(ang2*f)/(1+cos(ang2*f))
   om=(r2-r1)/2
   r=r2-om
   rmove 0 -om
   if ang/10=int(ang/10) then
      set lwidth thickl                            ! drawing every small circle fatter with: angular
   end if                                          ! distance to primitive = n*10deg
   arc r 90-ang 90+ang
   rmove 0 om
next ang

!drawing great circles in southern hemisphere
for ang=2 to 88 step 2                             ! defining the grid of great circles
   set lwidth thinl                                ! ang must not become 0 nor 90!
   ang2=180-ang
   r1=rp*sin(ang*f)/(1+cos(ang*f))
   r2=rp*sin(ang2*f)/(1+cos(ang2*f))
   om=(r2-r1)/2
   r=r2-om
   rmove 0 om
   if ang/10=int(ang/10) then
      set lwidth thickl                            ! drawing every small circle fatter with: angular
   end if                                          ! distance to primitive = n*10deg
   arc r 270-ang 270+ang
   rmove 0 -om
next ang

---

 

[Return to examples page]